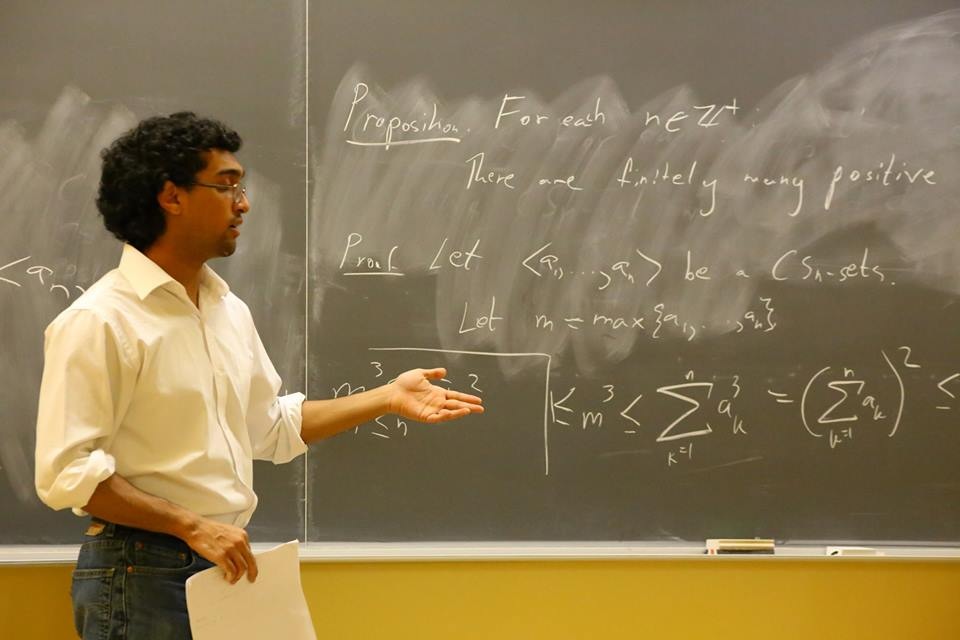Where Rigour Meets Intuition

Unlock the wonder of mathematics with an instructor who has over a decade of experience in mathematical education

NEWS: My series of textbooks, titled Rigorous Elementary Mathematics, is now available!

I can not stress enough how grateful I am for my daughter's math tutor, Samer. He has not only helped her improve her math skills, but has also ignited a genuine love and passion for the subject.
Click here to read all reviews
Ayla
Parent of a grade 9 Ontario high school student

Online Tutoring for Mathematics

Existsforall Academy is an online mathematics academy for high school and middle school students in Canada. If you are ready to invest in working with me on a one-on-one basis, then this is right for you. Please visit the Tutoring page, and fill in and submit the request form so that I can learn about your student and provide more information. Based on your student’s goals and current level of mathematical knowledge, I will develop an individualized study plan and custom homework sets to suit your student’s needs.

I teach students how to succeed while studying in various math courses and programs from grades 8 through 12. These include:

  • Ontario Grade 12: Calculus and Vectors
  • Ontario Grade 12: Advanced Functions
  • Ontario Grade 12: Data Management
  • Ontario Grade 11: Functions
  • Ontario Grade 10: Principles of Mathematics
  • Ontario Grade 9: Destreamed Mathematics
  • Ontario Grade 8: Mathematics
  • International Baccalaureate (IB) Mathematics (SL/HL, AA/AI) and pre-IB
  • Problem-solving to learn how to solve “thinking” questions

Due to the clarity and quality of my instruction, students generally obtain a level of mathematical understanding that they would find difficult to achieve independently. The academy does not offer official credit or exam invigilation.

Problem-Solving Principles

At every stage of being a student and teacher, I asked myself “Does math really have to be so hard?” and “How would I have trained my younger self?” From 2011 to 2021, I solved thousands of math problems, as a student and as an educator. The sources ranged from math contests and olympiads to university textbooks to classic puzzles. In the process, I learned how to tackle questions that I had never seen before, and I taught hundreds of students to do the same. I realized that the real problem was that I needed to not only obtain theoretical knowledge, but also needed to implement overarching strategies and tactics of problem-solving. If you would like to see a synthesis of the mentality that I internalized, sign up for my newsletter and I will email my Master List of Problem-Solving Principles to you! This document includes principles for psychological preparation, initial investigation, intuitive investigation, strategic and tactical rigorous investigation, analyzing a proof, and avoiding common mistakes. Don’t wait to receive these principles!