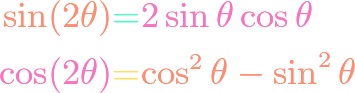Geometry

https://www.youtube.com/watch?v=PbrJHqBrSTk For sine and cosine, there are double and half angle trigonometric identities which we explore here. In particular, the cosine double angle identity has three forms, from among which we can choose one strategically depending on the situation. We prove these identities using the addition identities and the main Pythagorean identity.

https://www.youtube.com/watch?v=QfIvr-6xM-o The Pythagorean theorem is among the most famous theorems in math, with almost all school students studying it at some point. But how can we prove it? The Indian mathematician Bhaskara had a method that we modify and simpify here to prove the Pythagorean theorem and its converse.

https://www.youtube.com/watch?v=O_tg4X88HGE The angle bisector theorem gives us a remarkably simple relationship between the line segments that are produced by a cevian that is an angle bisector and the sides of a triangle. We discuss and prove this equation here.

https://www.youtube.com/watch?v=7y3gHO-Pz2o An incredible fact of Euclidean geometry is that the exterior angles of any convex polygon sum to $360^{circ}$, which is independent of the number of sides of the polygon. We prove this fact here using the formula for the sum of the interior angles of a convex $n$-gon.

A basic yet amazing fact of Euclidean geometry is that the sum of the interior angles of a convex polygon with $n$ sides is equal to $$180^{circ} (n-2),$$ which is a formula that depends only on $n$. We show how to prove this result by dissecting the polygon into triangles.